This is a special notes for all CAT and MBA aspirants.. by IIM Topper.
QUANT THEORY
1) nPr = n!/(n-r)!
2) nPn = n!
3) nCr = n!/(n-r)!r!
4) nCn = 1
5) nP0 = 1
6) nC0 = 1
7) AP An = a + (n-1)d
Sn = n/2[2a + (n-1)d]
GP An = ar(n-1)
Sn = a(rn – 1 )/ (r-1)
S∞ = a/(1-r)
9) 1 mile = 1760 yards
10) 1 yard = 3 feet
11) 1 mile2 = 640 acres
12) I gallon = 4 quarts
13) 1 quart = 2 pints
14) 1 pint = 2 cups
15) 1 cup = 8 ounces
16) 1 pound = 16 ounces
17) 1 ounce = 16 drams
18) 1 kg = 2.2 pounds
19) 30-60-90 triangle è 1:√3:2 sides
20) 45-45-90 triangle è 1:1:√2 sides
21) a3>b3 è a>b
22) If A than B => not B than not A
23) Zero divided by any nonzero integer is zero.
24) Division by 0 is undefined.
25)



26) The standard deviation is a statistic that tells you how tightly all the various examples are clustered around the mean in a set of data. When the examples are pretty tightly bunched together and the bell-shaped curve is steep, the standard deviation is small. When the examples are spread apart and the bell curve is relatively flat, that tells you have a relatively large standard deviation.
27) n(A U B U C) = n(A) + n (B)+ n(C) – n(A n B) – n(A n C) – n(B n C) + n(A n B n C)
28) n(Aonly) = n(A) – n(A n C) – n(A n B) + n(A U B U C)
29) Dividend = Divisor * Quotient + Remainder
30) LCM * HCF = Product of 2 numbers.
31) 1 + 2 + 3 ………………..n = n * n+1 / 2
32) Sum of squares of 1st n natural numbers = n (n+1)(2n+1) / 6
33) Sum of cubes of 1st n natural numbers = [n (n+1)/2]2
34)
Squares and Cubes
35)
Fractions and Percentage:
36) Average speed = Total distance / Total Time
When equal distances are covered in different speed then we take the harmonic mean
Av Speed = 2ab / a + b
Different distances in same time we take AM
Av Speed is = a + b / 2
37) Simple Interest: SI = PRT / 100, A = P + SI
38) 1 Nickel = 5 cents
1 dime = 10 cents
1 quarter = 25 cents
1 half = 50 cents
1 dollar = 100 cents
39) Equilateral triangle, Area = (√3 * a2)/4
40) Area of trapezium = ½ (Height * Sum of parallel sides)
41) Arc Length = (θ/ 360) 2 ∏ r
42) Area of sector = (θ/ 360) ∏ r2
43) Equal chords are equidistant from the center.
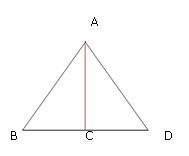
44) (x+y) 8 = 8C8x8 + 8C7x7y + 8C6x6y2 + 8C5x5y3 + … + 8C2x2y6 + 8C1xy7 + 8C0y8
45) Sometimes we get so involved with the nitty-gritties of mathematics that we start functioning like automatons and stop thinking. Don’t fall prey to this trap. For example, what is the probability that a number amongst the first 1000 positive integers is divisible by 8? Don’t start counting the multiples of 8! The figure of 1000 is a red herring. Use a little common sense. The numbers will be 8,16,24,32…So, 1 in every 8 numbers is a multiple of 8, even if you consider the first million integers. So Probability is 1/8
46) The number of integers from A to B inclusive is = B -A +1
47) Average of consecutive numbers:
Eg from 13 to 77 = (13+77)/2
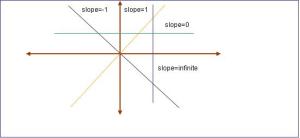
48) Slope = (change in y)/(change in x)
49) 00 = undefined
50)
51) Sum of interior angles of a polygon with n sides = (n-2)*180
52) Degree measure of one angle in a regular polygon with n sides
= {(n-2)*180 }/n
53) When multiplying or dividing both sides of an inequality by a negative number, the inequality sign reverses.
–x < y => -(-x) > y => x > -y
54) Fraction > (fraction)2 for all positive fractions
55) Fraction > √(fraction ) for all positive fractions
56) If n is a positive integer, (n6)/2 = √(n12 / 4)
57) If z1, z2, z3 … zn are consecutive positive integers and their average is an odd integer => n is odd => sum of series is odd
58) In a triangle with sides of measure a, b and c SHAPE \* MERGEFORMAT , a-b a + b = odd
59) Before confirming try and back solve and make sure that u have answered what has been asked.
60) When the question mentions prime number, remember to think of 2 too.
61) In a triangle, if the sum of two angles = third angle, then it is a right angled triangle.
62) Do not transport information from another statement unless considering both collectively.
63) A-b = odd => a + b = odd
64)
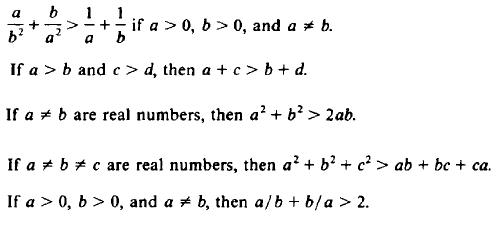
65)

66) If a DS question simply asks whether a, b, c and d are consecutive integers; use your brain. It has just asked u to answer if they are consecutive, not if they are consecutive in order.
67) Measure of an angle of a cyclic polygon = 180 – 360/n , where n is the number of sides of the polygon.
68) Sometimes, mistakes might also be committed by simply misreading the statement. Eg
Both Tim and Harry received an acre of land more than Neel => t = n + 1, h = n + 1
Tim and Harry received an acre more than Neel => t + h = n+1
69)
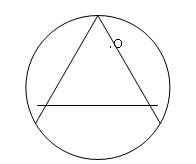
Let Triangle ABC be equilateral with each side of measure ‘a’ and AC ^ BD
ð AB = BD = AD = a
ð Ða = Ðb = Ðc = 600
ð AC = √(a2 + a/2 2)
= √3*a/2
ð Area = √3 * a2/4
ð Perimeter = 3a
ð Radius of circle O = a/√3 = AC * 2/3
Radius of circle O’ = √3a/6 = AC * 1/3
 70) Two
70) Two 
circles will touch each or intersect each other if the distance between their centers d is such that
R – r £ d £ R + r, where R and r are the radii of the two circles
71) Remainder of less than two means not just one; it also means remainder of zero.
72) Do not make unwarranted assumptions. 12 midnight to 12 noon does not mention what days, and hence you cannot find out the time period.
73) Standard deviation of a set is always negative and equals zero only if all elements of the set are equal.
74) If the difference between the largest and the smallest divisor of a number is X, the number is X + 1
75) Always remember the special watch out cases in DS questions. If the question mentions mean of a set, the mean can be ZERO also.
76) If area of a rectangle is known, diagonal is known, perimeter can be found
a2 + b2 = diagonal2
a2 + b2 + 2ab = diagonal2+ 2ab
(a + b)2 = diagonal2+ 2*area
77) √(y2) = |y| => y if y is positive, -y if y is negative
78) angle = mod [(60H - 11M) /2 ]
H = value of hour hand
M = value of minute hand
eg, if time is 2:30, then H =2 and M =30
79) Every number raised to power 5 has the number itself as unit digit
80) If a + b + c = Z, than the largest of a, b, and c cannot be greater than the mean of the other two.
81) The rule that one side of a triangle cannot be > sum of other two, only applies to sides, not angles
82) FINALLY, MAKE SURE OF WHAT THE QUESTION SAYS – INTEGER MEANS INTEGRAL LENGTH. And, DIVIDING A WIRE INTO PIECES, DOES NOT NECESSARILY IMPLY THAT THEY WILL BE INTEGRAL LENGTHS. Similarly, that a boat covers a distance upstream in 3 hours, states only the time, even if it has been mentioned that it covers a a distance 12 km downstream in 2 hours.
83) x2 = 9*y2 does not necessarily imply that x2 > y2. (Hint : consider x=y=0)
84) When we say multiples between 16 and 260, and inclusive/exclusive is not mentioned, take 16 and 260 to be exclusive.
85) The statement implies :
The hourly wage for each employee ranges from $5 an hour to $20 an hour.
minimum average = (20 + 5 + 5 + 5 + 5)/5
maximum average = (5 + 20 + 20 + 20 + 20)/5
Just mug up these notes and you will be able to crack any MBA exam like CAT,XAT,XLRI,FMS and GMAT.
QUANT THEORY
1) nPr = n!/(n-r)!
2) nPn = n!
3) nCr = n!/(n-r)!r!
4) nCn = 1
5) nP0 = 1
6) nC0 = 1
7) AP An = a + (n-1)d
Sn = n/2[2a + (n-1)d]
GP An = ar(n-1)
Sn = a(rn – 1 )/ (r-1)
S∞ = a/(1-r)
9) 1 mile = 1760 yards
10) 1 yard = 3 feet
11) 1 mile2 = 640 acres
12) I gallon = 4 quarts
13) 1 quart = 2 pints
14) 1 pint = 2 cups
15) 1 cup = 8 ounces
16) 1 pound = 16 ounces
17) 1 ounce = 16 drams
18) 1 kg = 2.2 pounds
19) 30-60-90 triangle è 1:√3:2 sides
20) 45-45-90 triangle è 1:1:√2 sides
21) a3>b3 è a>b
22) If A than B => not B than not A
23) Zero divided by any nonzero integer is zero.
24) Division by 0 is undefined.
25)



26) The standard deviation is a statistic that tells you how tightly all the various examples are clustered around the mean in a set of data. When the examples are pretty tightly bunched together and the bell-shaped curve is steep, the standard deviation is small. When the examples are spread apart and the bell curve is relatively flat, that tells you have a relatively large standard deviation.
27) n(A U B U C) = n(A) + n (B)+ n(C) – n(A n B) – n(A n C) – n(B n C) + n(A n B n C)
28) n(Aonly) = n(A) – n(A n C) – n(A n B) + n(A U B U C)
29) Dividend = Divisor * Quotient + Remainder
30) LCM * HCF = Product of 2 numbers.
31) 1 + 2 + 3 ………………..n = n * n+1 / 2
32) Sum of squares of 1st n natural numbers = n (n+1)(2n+1) / 6
33) Sum of cubes of 1st n natural numbers = [n (n+1)/2]2
34)
Squares and Cubes
| Number ( x ) | Square ( x 2 ) | Cube ( x 3 ) |
| 1 | 1 | 1 |
| 2 | 4 | 8 |
| 3 | 9 | 27 |
| 4 | 16 | 64 |
| 5 | 25 | 125 |
| 6 | 36 | 216 |
| 7 | 49 | - |
| 8 | 64 | - |
| 9 | 81 | - |
| 10 | 100 | - |
| 11 | 121 | - |
| 12 | 144 | - |
| 13 | 169 | - |
| 14 | 196 | - |
| 15 | 225 | - |
| 16 | 256 | - |
| 17 | 289 | |
| 18 | 324 | |
| 19 | 361 | |
| 21 | 441 | |
| 22 | 484 | |
| 23 | 529 | |
| 24 | 576 | |
| 25 | 625 |
Fractions and Percentage:
| Fraction | Decimal | Percentage |
| 1 / 2 | 0.5 | 50 |
| 1 / 3 | 0.33 | 33 1/3 |
| 2 / 3 | 0.66 | 66 2/3 |
| 1 / 4 | 0.25 | 25 |
| 3 / 4 | 0.75 | 75 |
| 1 / 5 | 0.2 | 20 |
| 2 / 5 | 0.4 | 40 |
| 3 / 5 | 0.6 | 60 |
| 4 / 5 | 0.8 | 80 |
| 1 / 6 | 0.166 | 16 2/3 |
| 5 / 6 | 0.833 | 83 2 / 3 |
| 1 / 8 | 0.125 | 12 1 / 2 |
| 3 / 8 | 0.375 | 37 1 / 2 |
| 5 / 8 | 0.625 | 62 1 / 2 |
| 7 / 8 | 0.875 | 87 1 / 2 |
| 1 / 9 | 0.111 | 11 |
| 2 / 9 | 0.222 | 22 |
| 1 / 10 | 0.1 | 10 |
| 1 / 20 | 0.05 | 5 |
| 1 / 100 | 0.01 | 1 |
36) Average speed = Total distance / Total Time
When equal distances are covered in different speed then we take the harmonic mean
Av Speed = 2ab / a + b
Different distances in same time we take AM
Av Speed is = a + b / 2
37) Simple Interest: SI = PRT / 100, A = P + SI
38) 1 Nickel = 5 cents
1 dime = 10 cents
1 quarter = 25 cents
1 half = 50 cents
1 dollar = 100 cents
39) Equilateral triangle, Area = (√3 * a2)/4
40) Area of trapezium = ½ (Height * Sum of parallel sides)
41) Arc Length = (θ/ 360) 2 ∏ r
42) Area of sector = (θ/ 360) ∏ r2
43) Equal chords are equidistant from the center.
44) (x+y) 8 = 8C8x8 + 8C7x7y + 8C6x6y2 + 8C5x5y3 + … + 8C2x2y6 + 8C1xy7 + 8C0y8
45) Sometimes we get so involved with the nitty-gritties of mathematics that we start functioning like automatons and stop thinking. Don’t fall prey to this trap. For example, what is the probability that a number amongst the first 1000 positive integers is divisible by 8? Don’t start counting the multiples of 8! The figure of 1000 is a red herring. Use a little common sense. The numbers will be 8,16,24,32…So, 1 in every 8 numbers is a multiple of 8, even if you consider the first million integers. So Probability is 1/8
46) The number of integers from A to B inclusive is = B -A +1
47) Average of consecutive numbers:
Eg from 13 to 77 = (13+77)/2
48) Slope = (change in y)/(change in x)
49) 00 = undefined
50)

51) Sum of interior angles of a polygon with n sides = (n-2)*180
52) Degree measure of one angle in a regular polygon with n sides
= {(n-2)*180 }/n
53) When multiplying or dividing both sides of an inequality by a negative number, the inequality sign reverses.
–x < y => -(-x) > y => x > -y
54) Fraction > (fraction)2 for all positive fractions
55) Fraction > √(fraction ) for all positive fractions
56) If n is a positive integer, (n6)/2 = √(n12 / 4)
57) If z1, z2, z3 … zn are consecutive positive integers and their average is an odd integer => n is odd => sum of series is odd
58) In a triangle with sides of measure a, b and c SHAPE \* MERGEFORMAT , a-b a + b = odd
59) Before confirming try and back solve and make sure that u have answered what has been asked.
60) When the question mentions prime number, remember to think of 2 too.
61) In a triangle, if the sum of two angles = third angle, then it is a right angled triangle.
62) Do not transport information from another statement unless considering both collectively.
63) A-b = odd => a + b = odd
64)
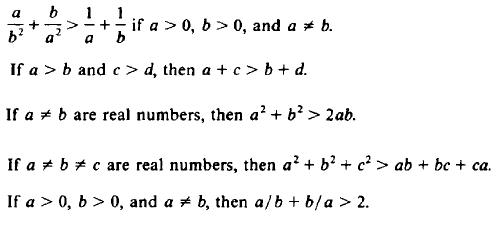
65)

66) If a DS question simply asks whether a, b, c and d are consecutive integers; use your brain. It has just asked u to answer if they are consecutive, not if they are consecutive in order.
67) Measure of an angle of a cyclic polygon = 180 – 360/n , where n is the number of sides of the polygon.
68) Sometimes, mistakes might also be committed by simply misreading the statement. Eg
Both Tim and Harry received an acre of land more than Neel => t = n + 1, h = n + 1
Tim and Harry received an acre more than Neel => t + h = n+1
69)

Let Triangle ABC be equilateral with each side of measure ‘a’ and AC ^ BD
ð AB = BD = AD = a
ð Ða = Ðb = Ðc = 600
ð AC = √(a2 + a/2 2)
= √3*a/2
ð Area = √3 * a2/4
ð Perimeter = 3a
ð Radius of circle O = a/√3 = AC * 2/3
Radius of circle O’ = √3a/6 = AC * 1/3
 70) Two
70) Two 
circles will touch each or intersect each other if the distance between their centers d is such that
R – r £ d £ R + r, where R and r are the radii of the two circles
71) Remainder of less than two means not just one; it also means remainder of zero.
72) Do not make unwarranted assumptions. 12 midnight to 12 noon does not mention what days, and hence you cannot find out the time period.
73) Standard deviation of a set is always negative and equals zero only if all elements of the set are equal.
74) If the difference between the largest and the smallest divisor of a number is X, the number is X + 1
75) Always remember the special watch out cases in DS questions. If the question mentions mean of a set, the mean can be ZERO also.
76) If area of a rectangle is known, diagonal is known, perimeter can be found
a2 + b2 = diagonal2
a2 + b2 + 2ab = diagonal2+ 2ab
(a + b)2 = diagonal2+ 2*area
77) √(y2) = |y| => y if y is positive, -y if y is negative
78) angle = mod [(60H - 11M) /2 ]
H = value of hour hand
M = value of minute hand
eg, if time is 2:30, then H =2 and M =30
79) Every number raised to power 5 has the number itself as unit digit
80) If a + b + c = Z, than the largest of a, b, and c cannot be greater than the mean of the other two.
81) The rule that one side of a triangle cannot be > sum of other two, only applies to sides, not angles
82) FINALLY, MAKE SURE OF WHAT THE QUESTION SAYS – INTEGER MEANS INTEGRAL LENGTH. And, DIVIDING A WIRE INTO PIECES, DOES NOT NECESSARILY IMPLY THAT THEY WILL BE INTEGRAL LENGTHS. Similarly, that a boat covers a distance upstream in 3 hours, states only the time, even if it has been mentioned that it covers a a distance 12 km downstream in 2 hours.
83) x2 = 9*y2 does not necessarily imply that x2 > y2. (Hint : consider x=y=0)
84) When we say multiples between 16 and 260, and inclusive/exclusive is not mentioned, take 16 and 260 to be exclusive.
85) The statement implies :
The hourly wage for each employee ranges from $5 an hour to $20 an hour.
minimum average = (20 + 5 + 5 + 5 + 5)/5
maximum average = (5 + 20 + 20 + 20 + 20)/5
Just mug up these notes and you will be able to crack any MBA exam like CAT,XAT,XLRI,FMS and GMAT.
Source:- http://catindiaonline.wordpress.com